Решите систему неравенств:
а) $\begin{equation*} \begin{cases} 2(x - 1) - 3(x - 2) < x &\\ 6x - 3 < 17 - (x - 5) & \end{cases} \end{equation*}$;
б) $\begin{equation*} \begin{cases} 3,3 - 3(1,2 - 5x) > 0,6(10x + 1) &\\ 1,6 - 4,5(4x - 1) < 2x + 26,1 & \end{cases} \end{equation*}$;
в) $\begin{equation*} \begin{cases} 5,8(1 - a) - 1,8(6 - a) < 5 &\\ 8 - 4(2 - 5a) > -(5a + 6) & \end{cases} \end{equation*}$;
г) $\begin{equation*} \begin{cases} x(x - 1) - (x^2 - 10) < 1 - 6x &\\ 3,5 - (x - 1,5) < 6 - 4x & \end{cases} \end{equation*}$.

Получай решения и ответы с помощью нашего бота

Решение

reshalka.com

ГДЗ учебник по алгебре 8 класс Макарычев. 35. Решение систем неравенств с одной переменной. Номер №886

Решение а

$\begin{equation*} \begin{cases} 2(x - 1) - 3(x - 2) < x &\\ 6x - 3 < 17 - (x - 5) & \end{cases} \end{equation*}$
$\begin{equation*} \begin{cases} 2x - 2 - 3x + 6 < x &\\ 6x - 3 < 17 - x + 5 & \end{cases} \end{equation*}$
$\begin{equation*} \begin{cases} 2x - 3x - x < 2 - 6 &\\ 6x + x < 17 + 3 + 5 & \end{cases} \end{equation*}$
$\begin{equation*} \begin{cases} -2x < -4 &\\ 7x < 25 & \end{cases} \end{equation*}$
$\begin{equation*} \begin{cases} x > 2 &\\ x < 3\frac{4}{7} & \end{cases} \end{equation*}$
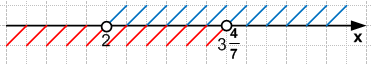
$2 < x < 3\frac{4}{7}$
$x ∈ (2;3\frac{4}{7})$
Решение рисунок 1

Решение б

$\begin{equation*} \begin{cases} 3,3 - 3(1,2 - 5x) > 0,6(10x + 1) &\\ 1,6 - 4,5(4x - 1) < 2x + 26,1 & \end{cases} \end{equation*}$
$\begin{equation*} \begin{cases} 3,3 - 3,6 + 15x > 6x + 0,6 &\\ 1,6 - 18x + 4,5 < 2x + 26,1 & \end{cases} \end{equation*}$
$\begin{equation*} \begin{cases} 15x - 6x > 0,6 - 3,3 + 3,6 &\\ -18x - 2x < 26,1 - 1,6 - 4,5 & \end{cases} \end{equation*}$
$\begin{equation*} \begin{cases} 9x > 0,9 &\\ -20x < 20 & \end{cases} \end{equation*}$
$\begin{equation*} \begin{cases} x > 0,1 &\\ x > -1 & \end{cases} \end{equation*}$
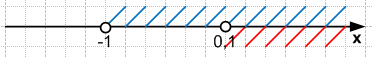
x > 0,1
x ∈ (0,1;+∞)
Решение рисунок 1

Решение в

$\begin{equation*} \begin{cases} 5,8(1 - a) - 1,8(6 - a) < 5 &\\ 8 - 4(2 - 5a) > -(5a + 6) & \end{cases} \end{equation*}$
$\begin{equation*} \begin{cases} 5,8 - 5,8a - 10,8 + 1,8a < 5 &\\ 8 - 8 + 20a > -5a - 6 & \end{cases} \end{equation*}$
$\begin{equation*} \begin{cases} -5,8a + 1,8a < 5 - 5,8 + 10,8 &\\ 20a + 5a > -6 & \end{cases} \end{equation*}$
$\begin{equation*} \begin{cases} -4a < 10 &\\ 25a > -6 & \end{cases} \end{equation*}$
$\begin{equation*} \begin{cases} a > -2,5 &\\ a > -0,24 & \end{cases} \end{equation*}$
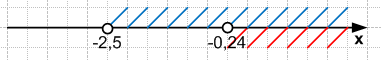
a > −0,24
a ∈ (−0,24;+∞)
Решение рисунок 1

Решение г

$\begin{equation*} \begin{cases} x(x - 1) - (x^2 - 10) < 1 - 6x &\\ 3,5 - (x - 1,5) < 6 - 4x & \end{cases} \end{equation*}$
$\begin{equation*} \begin{cases} x^2 - x - x^2 + 10 < 1 - 6x &\\ 3,5 - x + 1,5 < 6 - 4x & \end{cases} \end{equation*}$
$\begin{equation*} \begin{cases} -x + 6x < 1 - 10 &\\ -x + 4x < 6 - 3,5 - 1,5 & \end{cases} \end{equation*}$
$\begin{equation*} \begin{cases} 5x < -9 &\\ 3x < 1 & \end{cases} \end{equation*}$
$\begin{equation*} \begin{cases} x < -1,8 &\\ x < \frac{1}{3} & \end{cases} \end{equation*}$
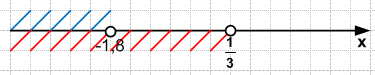
x < −1,8
x ∈ (−∞;−1,8)
Решение рисунок 1