Решите графически систему уравнений
$\begin{equation*}
\begin{cases}
ax + 3y = 11, &\\
5x + 2y = 12, &
\end{cases}
\end{equation*}$
если известно, что первое уравнение этой системы обращается в верное равенство при x = 5 и y = −3.
$\begin{equation*}
\begin{cases}
ax + 3y = 11 &\\
5x + 2y = 12 &
\end{cases}
\end{equation*}$
5a + 3 * (−3) = 11
5a − 9 = 11
5a = 11 + 9
a = 20 : 5
a = 4
$\begin{equation*}
\begin{cases}
4x + 3y = 11 &\\
5x + 2y = 12 &
\end{cases}
\end{equation*}$
$\begin{equation*}
\begin{cases}
3y = -4x + 11 &\\
2y = -5x + 12 &
\end{cases}
\end{equation*}$
$\begin{equation*}
\begin{cases}
y = -\frac{4}{3}x + \frac{11}{3} &\\
y = -\frac{5}{2}x + 6 &
\end{cases}
\end{equation*}$
$y = -\frac{4}{3}x + \frac{11}{3}$
$y = -\frac{5}{2}x + 6$
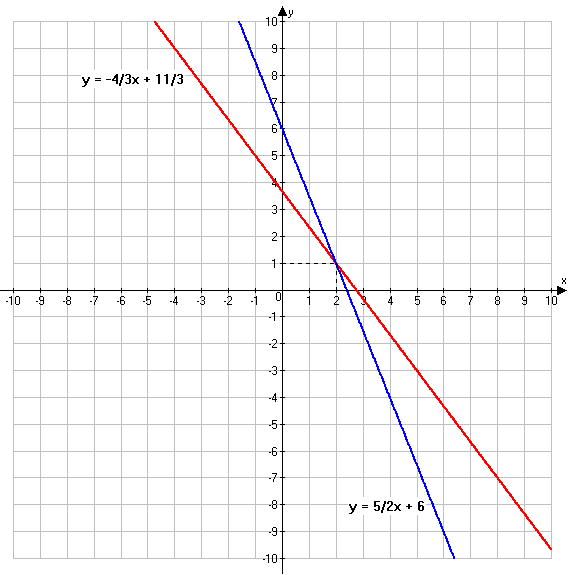
Ответ: (2;1)
Пожаулйста, оцените решение
vsebot.ru - Твоя нейросеть на базе СhatGPT, Gemini и др. Бесплатно навсегда!