Определите графически количество решений системы уравнений:
1)
$\begin{equation*} \begin{cases} xy = -1 &\\ x + 3y = 0 & \end{cases} \end{equation*}$
2)
$\begin{equation*} \begin{cases} xy = -1 &\\ x - 3y = 0 & \end{cases} \end{equation*}$
3)
$\begin{equation*} \begin{cases} xy = 6 &\\ 3x - 2y = 6 & \end{cases} \end{equation*}$

Получай решения и ответы с помощью нашего бота

Решение

reshalka.com

ГДЗ учебник по алгебре 8 класс Мерзляк, Полонский, Якир. §10. Упражнения. Номер №335

Решение 1

$\begin{equation*} \begin{cases} xy = -1 &\\ x + 3y = 0 & \end{cases} \end{equation*}$
$\begin{equation*} \begin{cases} y = -\frac{1}{x} &\\ 3y = -x & \end{cases} \end{equation*}$
$\begin{equation*} \begin{cases} y = -\frac{1}{x} &\\ y = -\frac{x}{3} & \end{cases} \end{equation*}$

$y = -\frac{1}{x}$
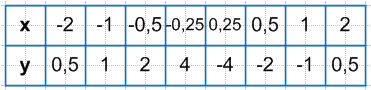
Решение рисунок 1

$y = -\frac{x}{3}$
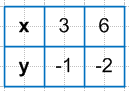
Решение рисунок 2

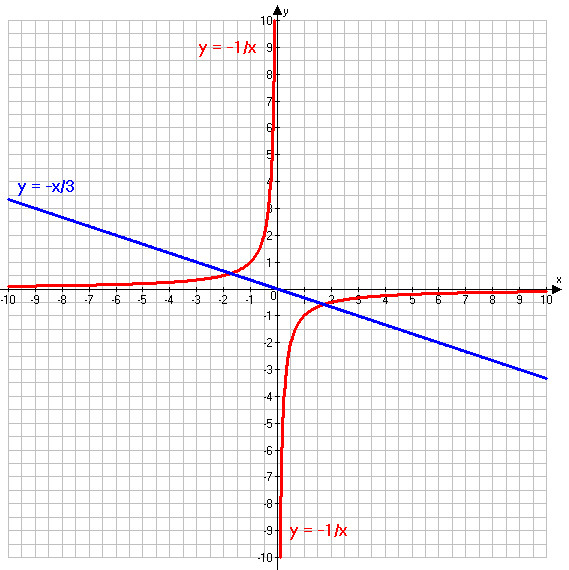
Решение рисунок 3
Ответ: система уравнений имеет 2 решения

Решение 2

$\begin{equation*} \begin{cases} xy = -1 &\\ x - 3y = 0 & \end{cases} \end{equation*}$
$\begin{equation*} \begin{cases} y = -\frac{1}{x} &\\ 3y = x & \end{cases} \end{equation*}$
$\begin{equation*} \begin{cases} y = -\frac{1}{x} &\\ y = \frac{x}{3} & \end{cases} \end{equation*}$

$y = -\frac{1}{x}$
Решение рисунок 1

$y = \frac{x}{3}$
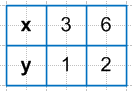
Решение рисунок 2

Решение рисунок 3
Ответ: система уравнений не имеет решений

Решение 3

$\begin{equation*} \begin{cases} xy = 6 &\\ 3x - 2y = 6 & \end{cases} \end{equation*}$
$\begin{equation*} \begin{cases} y = \frac{6}{x} &\\ 2y = 3x - 6 & \end{cases} \end{equation*}$
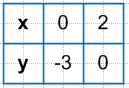
$\begin{equation*} \begin{cases} y = \frac{6}{x} &\\ y = 1,5x - 3 & \end{cases} \end{equation*}$

$y = \frac{6}{x}$
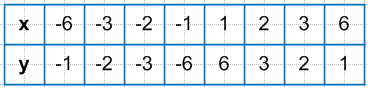
Решение рисунок 1

y = 1,5x − 3
Решение рисунок 2

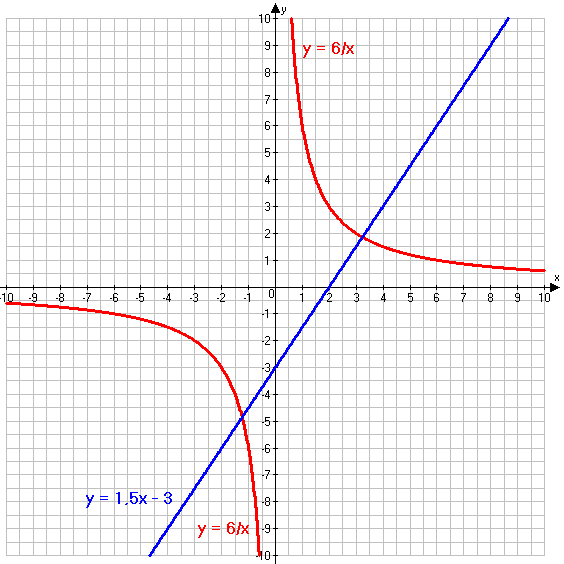
Решение рисунок 3
Ответ: система уравнений имеет 2 решения