ГДЗ Алгебра 8 класс Макарычев, Миндюк, Нешков, Суворова, 2013

Авторы: Макарычев, Миндюк, Нешков, Суворова.

Издательство: "Просвещение" 2013 г

Постройте график функции, заданной формулой:
а) $y = \frac{\sqrt{x}}{x}$;
б) $y = \frac{-2\sqrt{x^2}}{x}$;
в) $y = x\sqrt{x^2}$;
г) $y = -x\sqrt{x^2}$.

Получай решения и ответы с помощью нашего бота

Решение

reshalka.com

ГДЗ учебник по алгебре 8 класс Макарычев. К параграфу 6. Номер №485

Решение а

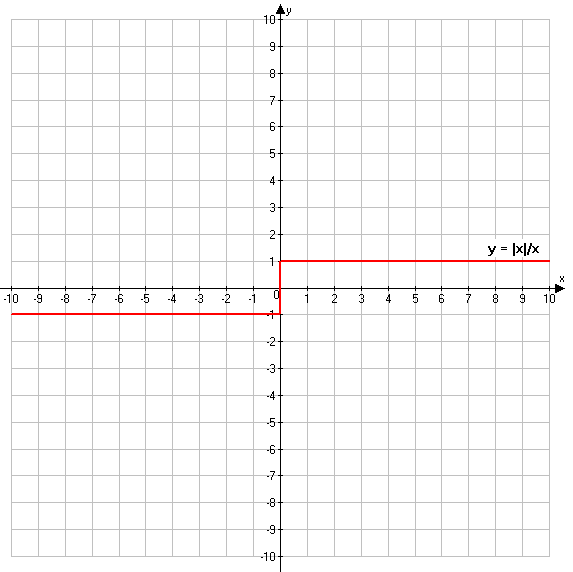
$y = \frac{\sqrt{x}}{x} = \frac{|x|}{x}$
$\begin{equation*} \begin{cases} 1, x > 0 &\\ -1, x < 0 & \end{cases} \end{equation*}$
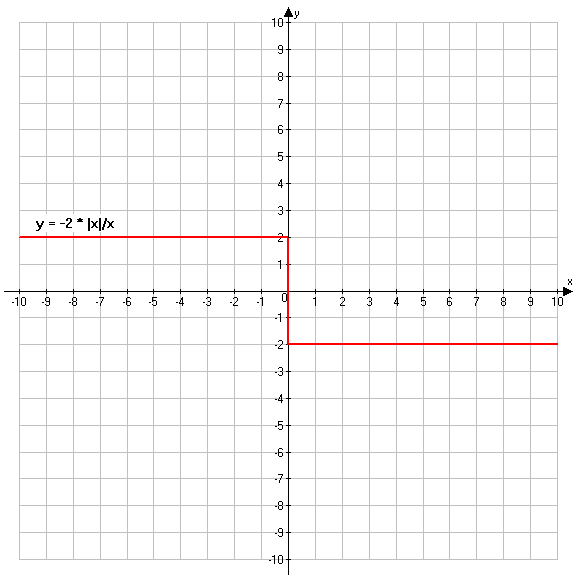
Решение рисунок 1

Решение б

$y = \frac{-2\sqrt{x^2}}{x} = -2\frac{|x|}{x}$
$\begin{equation*} \begin{cases} -2, x > 0 &\\ 2, x < 0 & \end{cases} \end{equation*}$
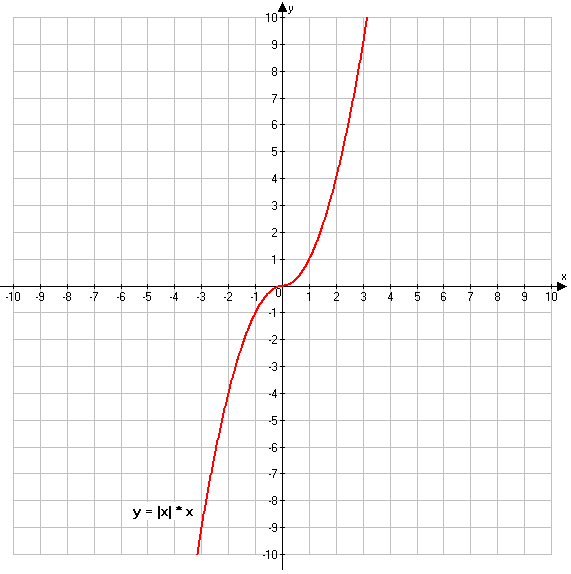
Решение рисунок 1

Решение в

$y = x\sqrt{x^2} = x * |x|$
$\begin{equation*} \begin{cases} x^2, x ≥ 0 &\\ -x^2, x < 0 & \end{cases} \end{equation*}$
Решение рисунок 1

Решение г

$y = -x\sqrt{x^2} = -x * |x|$
$\begin{equation*} \begin{cases} -x^2, x ≥ 0 &\\ x^2, x < 0 & \end{cases} \end{equation*}$
Решение рисунок 1